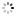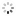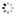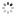Important Modern & Vintage Timepieces
New York, Apr 15, 2015
LOT 81
DE BETHUNE PIECE UNIQUE TOURBILLON MINUTE REPEATER PERPETUAL CALENDAR & EQUATION OF TIME PINK GOLD De Bethune, "Pièce Unique, Tourbillon, Rèpètion à Minutes, Equation du Temps, Quantieme Perpetuel". Made in 2002. Extremely fine and unique, large, minute-repeating, 18K pink gold manual-winding wristwatch with one-minute tourbillion regulator, perpetual calendar, equation of time and an 18K pink gold De Bethune buckle. Accompanied by a technical description and by a certificate of authenticity.
USD 60,000 - 120,000
HKD 465,000 - 930,000 / CHF 60,000 - 120,000
Sold: USD 93,750
Three-body, solid, polished, curved bezel, conical lugs, flat band with date corrector at 2, octagonal crown, transparent snap-on case back, sapphire crystals. Satiné and hand-guilloche silver with painted radial Roman numerals, outer minute track, the center with equation of time graduations in the upper half, apertures for the date between 7 and 8 and the months in Roman numerals between 4 and 5. Blued steel Breguet hands, pink gold sun equation of time hand. Rhodium plated with cotes de Genève decoration, 20 jewels, wolf's tooth winding, lateral lever escapement, monometallic balance adjusted to 5 positions, self-compensating Breguet balance spring, A-form polished steel tourbillion carriage with index regulator, brushed steel bridge, repeating on gongs activated by a slide on the band.
| Grading System | |
|---|---|
Grade: |
|
Case: 2 |
Very good |
Movement: 1* |
As new Overhaul recommended, at buyer's expense |
Dial: 1-01 |
As new HANDS Original |