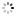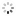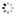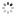Important Watches
Geneva, Mar 20, 2010
LOT 180
Fatio Junod Pivoted Detent Chronometer with Equation of Time Chart Fatio Junod a Genève, No. 1907. Made circa 1860. Very fine and very rare, 18K gold pocket chronometer with pivoted detent and equation of time chart.
CHF 6,500 - 9,000
USD 6,000 - 8,300 / EUR 4,500 - 6,200
C. Four-body, ?bassine et filets?, polished. Hinged gold cuvette engraved with equation of time chart showing the average time variation at midday for each month of the year for the 1st and 15th day of the month. D. Silver with applied gold radial Roman numerals, outer dot minute divisions, subsidiary seconds, foliate and floral engraved center. Blued steel spade hands. M. 47 mm., matte pink gilt, fusee and chain, maintaining power, 10 jewels, pivoted detent chronometer escapement with jeweled locking stone, bimetallic compensation balance with gold timing screws, steel balance spring with terminal curve and unusual stud fastening, index regulator. Cuvette signed. Diam. 53 mm.
| Grading System | |
|---|---|
Grade: AAA |
Excellent |
Case: 3 |
Good |
Movement: 3* |
Good Overhaul recommended, at buyer's expense |
Dial: 3-6-01 |
Good Slightly oxidized HANDS Original |
Notes
Equation of time The difference between the true solar day and the mean solar day (or time told by a clock or watch). It has two major causes, the first being the inclination of the Earth's Equator and the second being the elliptical course of the Earth around the Sun. Equation of Time due to obliquity (the Earth's tilt). If the Earth's rotational axis were not tilted with respect to its orbit around the Sun, the apparent motion of the Sun along the Ecliptic would fall directly on the Equator, covering the same angles along the Equator in equal time. However, the angular movement is not linear in terms of time because it changes as the Sun moves above and below the Equator. The projection of the Sun's motion onto the Equator will be at a maximum when its motion along the Ecliptic is parallel to the Equator (at the summer and winter solstices) and will be at a minimum at the equinoxes.
Equation of Time due to Unequal Motion (the Earth's elliptical orbit). The orbit of the Earth around the Sun is an ellipse. The distance between the Earth and the Sun is at a minimum around December 31 and is greatest around July 1. The Sun's apparent longitude changes fastest when the Earth is closest to the Sun. The Sun will appear on the meridian at noon on these two dates and the Equation of Time due to unequal motion will then be zero. The mean solar day, calculated by averaging all the days of the year, was invented by astronomers for convenience so that the day would always be 24 hours. True solar time and mean solar time coincide four times a year, on April 16, June 14, September 1, and December 25. On these days, the equation will equal zero. On the other 361 days, the equation of time must be used to indicate the difference between solar and mean time, amounting over 16 minutes at certain times of year. The minimum difference occurs on November 1 with a loss of 16 minutes and 23 seconds, and the maximum occurs on February 11 with an increase of 14 minutes 20 seconds. This positive and negative value is offset in the time of the local noon and those of sunrise and sunset. Equation of time, often represented by a figure eight, called an ?analemma?, can be approximated by the following formula: E = 9.87 * sin (2B) ? 7.53 * cos (B) ? 1.5 * sin (B) Where: B = 360 * (N-81) / 365 Where: N = day number, January 1 = day 1.